Webinar Frequently Asked Questions

Q: How friendly is the program for children with learning disabilities?
Q: How can the Universal Design for Learning method of teaching be used with this program? 

A: We use guidelines from the Universal Design for Learning as a framework to provide suggestions for teacher moves that will open up access to the on-grade-level mathematics for a wide range of learners.

Math language routines help all students learn content and the language of mathematics simultaneously. The curriculum gives prompts for teacher moves that help each student to find their voice in a classroom where learning is driven by student discourse. For each grade level course K–12, each lesson of the curriculum has a suggested strategy for access for students with disabilities and for English learners in the teacher lesson plan.

When a teacher is responding to student work during the lesson, each student’s learning is being addressed just in time. Teachers can take advantage of built-in features of the curriculum to respond to student thinking during grade-level instruction, including:

• accessible tasks designed to invite a variety of responses and give students a chance to learn from studying multiple approaches
• warm-up activities and activity launches designed to provide an invitation to the mathematics and activate prior knowledge in the service of accessing grade-level content
• teacher guidance in each activity to advance student thinking (K–5) and address misconceptions (6–12)
• Centers in IM K–5 Math™ 
• Algebra 1 Extra Supports materials
• suggested adjustments based on student responses to cool-downs (IM K–12 Math™)
• pre-unit diagnostic problems (included in IM 6–12 Math™ as a Check Your Readiness assessment, and in IM K–5 Math™ as labeled practice problems).

Q: Do you offer pre and post assessments? What data should we be collecting during instruction?

A: Teachers play a critical role in mediating student learning in a problem-based classroom. Teachers continually assess student understanding by monitoring students’ work during the lesson, through the use of daily formative assessments (cool-downs), and additional practice opportunities such as centers, practice problems, and assessments. 

Illustrative Mathematics uses an asset based approach to student understanding and by the purposeful design of tasks that offer multiple entry points and solution paths, all students are able to engage in grade-level mathematics. Teachers support access through the use of routines such as the 5 Practices for Orchestrating Productive Mathematics Discussions and by the use of purposeful questions that advance and assess student understanding. 

When a teacher is responding to student work during the lesson, each student’s learning is being addressed just in time. Teachers can take advantage of built-in features of the curriculum to respond to student thinking during grade-level instruction, including:

• accessible tasks designed to invite a variety of responses and give students a chance to learn from studying multiple approaches
• warm-up activities and activity launches designed to provide an invitation to the mathematics and activate prior knowledge in the service of accessing grade-level content
• teacher guidance in each activity to advance student thinking (K–5) and address misconceptions (6–12)
• Centers in IM K–5 Math™ 
• Algebra 1 Extra Supports materials
• suggested adjustments based on student responses to cool-downs (IM K–12 Math™)
• pre-unit diagnostic problems (included in IM 6–12 Math™ as a Check Your Readiness assessment, and in IM K–5 Math™ as labeled practice problems).

In IM 6–12 Math, the authors chose to include pre-unit diagnostic assessments rather than a beginning of the year assessment. The data from those Check Your Readiness assessments provide teachers with actionable data to support student access to upcoming lessons in the unit.

Q: Do you have any tips to support coherence with block scheduling? (A day B day model)

A: This is a link to a video that explains our IM Resource Hub where you can find additional resources for implementation, including block scheduling guidance. Additionally, you might want to review the IM Certified blog post Planning Lessons for a Block Schedule.

Q: When and what is the best way to introduce math center work?
Q: How should centers be organized?

A: Center organization is unique to individual teachers. This IM Certified blog post, Making IM Centers Work, provides guidance on setting up centers for the first time and offers examples of how different teachers organize centers in their classrooms. 

Q: Does IM plan to include more culturally relevant math activities into the curriculum?

A: The IM authoring team is constantly seeking feedback and revising our curriculum for future versions. Please share your specific feedback directly with the IM curriculum team using this form.

If you wish to learn more about existing culturally responsive pedagogy, we invite you to explore these additional resources:  

• IM K–5 Math as a Support for Culturally Responsive Pedagogy webinar
• Supporting Culturally Responsive Pedagogy with IM K–5 Math™ blog
• Culturally Responsive Teaching and Math blog
• K–5 Curriculum Design Features that Support Equity and Inclusion blog
• Making Sense of Story Problems blog

Q: How much do you need to supplement the IM curriculum with your own materials?

A: IM K–12 Math^TM is a complete, standards-aligned curriculum with consistency in lesson structure and design for a problem-based approach that is driven by student discourse. 

Q: How do you differentiate the material in this curriculum?
Q: What does differentiation look like in a successfully responsive IM classroom?

A: IM’s problem-based approach supports a positive, inclusive classroom culture and provides both access and challenge for diverse learners. IM K–12 Math provides multiple entry points and is designed to balance access and challenge. We invite you to explore the following features in the curriculum that support students with multiple entry points:

• design principles
• launch, activity, and synthesis for each activity
• Instructional Routines (including the 5 Practices for Orchestrating Productive Mathematics Discussions)
• math language routines
• access for students with disabilities  
• access for english learners
• advancing student thinking
• responding to student thinking (component of cool-downs)
• centers (in IM K–5 Math)
• section-level practice problems (in IM K–5 Math) include pre-unit problems, problems aligned to grade-level lessons, and exploration problems

Individual students can have their unique needs addressed within the structure of the IM lessons and centers. When a teacher is responding to student work during the lesson, each student’s learning is being addressed just in time by the teacher’s usage of advancing and assessing questions. Each lesson of the curriculum has a suggested strategy for access for diverse learners. 

In addition, the centers in IM K–5 math are intended to give students time to practice skills and concepts that are developed across the year. There are 2 types of centers. Addressing centers address the work of a lesson or section of a unit. Supporting centers review prior unit or prior grade-level understandings and fluencies. 

“Are You Ready For More?” extension activities in each IM 6–12 Math lessons offer additional opportunities for challenge.

Q: What is the best approach to engaging students who traditionally may have been able to disengage from the learning? What is the best way to invite them into the discussions? Is it just a matter of time and opportunity?

A: IM K–12 Math^TM uses a problem-based approach that is driven by student discourse. (Visit our current Certified Partners’ sites—Kendall Hunt, Imagine Learning, or McGraw-Hill—for the full curriculum.) Students should take an active role, both individually and in groups, to see what they can figure out before having things explained to them or being told what to do. Teachers play a critical role in mediating student learning, but that role looks different than simply showing, telling, and correcting. The teacher’s role is to:

1. ensure students understand the context and what is being asked
2. ask questions to advance students’ thinking in productive ways
3. help students share their work and understand others’ work through orchestrating productive discussions
4. synthesize the learning with students at the end of activities and lessons

The IM teacher guides offer suggestions for grouping students to promote collaboration and teacher moves to advance student thinking.

 An expectation is that teachers will start off the school year establishing norms and building a mathematical community where all learners are engaged in thinking, learning, and communicating their mathematical ideas. In a mathematical community, all students have the opportunity to express their mathematical ideas and discuss them with others, which encourages collective learning and high engagement. The curriculum is a resource to support equitable structures and practices, which ensure all students have access to grade-level content and provides teachers with guidance to listen to, learn from, and support each student. 

Q: Where should teachers go for enrichment/reteach materials?

A: See Differentiation.

Q: What materials do you offer to use with parents who think the instruction is happening “too slowly” when compared to more traditional approaches?

A: These blog posts and resources might be helpful in partnering with families to better understand IM K–12 Math^TM:

Blog posts:

• Building A Supportive Home/School Partnership (9–12 Focus)
• Building a Supportive Home/School Partnership (6–8 Focus)
• FASTalk: Activating the Power of Families to Support Mathematics

Information for families is available in the partner platforms including: 

• Unit Family Support Resources with Family Support Letters that outline the work of the unit 
• Family Guide for K–5 Instructional Routines located on the IM Resource Hub
• In Grades K–5, each family unit letter also includes a section labeled “Try it at Home” with math prompts and questions for families to interact with their child at home.
• For Grade 6–Algebra 1, Family Support Materials also include videos of the summaries for each section of a unit. Grant funding made this project possible for Grade 6–Algebra 1, but we don’t currently have the videos for K–5, Geometry, or Algebra 2 courses.
• In IM 6–12 Math^TM, each student lesson includes activities that are followed by a student lesson summary. An example from the Algebra 1 course can be found at this link: (You’ll need to scroll down the page. Visit our current Partners’ sites—Kendall Hunt, Imagine Learning, or McGraw-Hill—for the full curriculum). 

Q: How is fluency addressed in Illustrative Mathematics? Are there enough iterations for students to solidify fluency skills?

A: We created this fluency website that describes how we approach fluency in IM K–5 Math.

Other helpful links include multiple blog posts by our authors:

• The Joy of Fluency
• Fluency Development Within and Across the Grades in IM K–5 Math part 1: Addition and Subtraction
• Fluency Development Within and Across the Grades in IM K–5 Math part 2: Addition and Subtraction
• Fluency Development Within and Across the Grades in IM K–5 Math part 3: Multiplication and Division
• Fluency Development Within and Across the Grades in IM K–5 Math part 4: Multiplication and Division

Q: Have you heard of “doubles plus one” strategies? Are these specific strategies reinforced in IM K–5 Math^TM?

A: IM K–2 Math^TM activities and centers allow students to explore and notice fact relationships and provide opportunities for students to reflect on facts they know and those that are not known yet. When reflecting on this shift in approach, teachers often make comments such as this one by a school math coach: “We trusted the IM curriculum and didn’t supplement by teaching strategies used in our old curriculum. It is quite stunning the way students described and used strategies that worked for them!”

Q: How do teachers build fact fluency in middle and high school using IM?

A: Fact fluency is not a focus for IM 6–12 Math^TM as it is a focus in the grade-level standards for IM K–5 Math^TM. However, developing procedural fluency is an integral part of the curriculum design. Each unit begins with a pre-assessment that helps teachers gauge what students know about both prerequisite and upcoming concepts and skills, so that teachers can gauge where students are and make adjustments accordingly. The initial lesson in a unit is designed to activate prior knowledge and provide an easy entry point to new concepts, so that students at different levels of both mathematical and English language proficiency can engage productively in the work. As the unit progresses, students are systematically introduced to representations, contexts, concepts, language, and notation. As their learning progresses, they make connections between different representations and strategies, consolidating their conceptual understanding, and see and understand more efficient methods of solving problems, supporting the shift towards procedural fluency. The distributed practice problems give students ongoing practice, which also supports developing procedural proficiency.

You may wish to read more about procedural fluency in IM 6–12 Math^TM in this IM Certified Blog post, Developing Conceptual Understanding and Procedural Fluency.

Q: How do I start? Where do I start?
Q: We are going to be using IM next school year,  so I am wondering if you could tell me something that I could focus on first.
Q: Our school is considering adopting IM in our K–5 classrooms. Are there any suggestions for teachers?
Q: What should the focus be for the first year of implementation?
Q: What are the most important parts of your program that a first-year teacher using it should know?

A: The instructional routines in IM K–12 Math^TM are a great place to start. In fact, many teachers find it helpful to try out some of the instructional routines even before implementing IM K–12 Math^TM. Many of the instructional routines are familiar to teachers who might already be using them in the classroom. Examples include Which One Doesn’t Belong, Notice and Wonder, Number Talks, and the 5 Practices for Orchestrating Productive Mathematics Discussions. You can see a full list of instructional routines on our site under “How to Use the Materials.”

The instructional routines are also one of our IM Certified Professional Learning offerings offered in preparation for IM implementation.

Q: How is assessment and grading handled?

A: When we created the curriculum, we chose not to prescribe homework assignments or decide which student work should count as a graded event. This was deliberate because homework policies and grading practices are highly variable, localized, and values-driven shared understandings that evolve over time. For example, the curriculum needed to work for schools where nightly, graded assignments are expected; schools where no work done outside of class is graded; and schools who take a feedback-only approach for any formative work.

The IM Certified Blog post, IM 6–12 Math: Grading and Homework Policies and Practices, shares some information gathered from a survey sent to teachers using IM in their classrooms. 

Q: Do teachers know when kids should master concepts based on the scope and sequence of the curriculum?

A: It is a district decision to define how they will assess for “mastery.” The standards are intended to be learned by the end of the school year. This is particularly important to understand regarding fluency standards. There aren’t specific times where “mastery” is expected prior to the end of the year within IM K–12 Math^TM. 

A great way to monitor student understanding is by monitoring their progress toward section goals and what evidence can be used to determine understanding of those goals.

Q: What are IM’s partners? 

A: The three IM Certified curriculum partners—Imagine Learning (K–12), Kendall Hunt (K–12), and McGraw-Hill Education (6–12)—exclusively offer the IM Certified curricula for free or via enhanced teacher and student experiences in digital and print formats. IM Certified partners make an agreement that they will not change or modify the curriculum from the original design, and they receive updates to the curriculum prior to public release. Having three partners for IM 6–12 Math^TM and two partners for IM K–5 Math^TM gives districts and schools more choices and flexibility to meet the needs of their students.

Q: What’s the best way to launch IM as a new adoption?

A: Implementation success increases when teachers and school leaders share a vision for mathematics teaching and learning, understand what this may mean in terms of their practice, and understand why and how adopting IM is a part of that vision. Many schools find it helpful to begin by having a discussion regarding what your district vision is for math instruction. NCTM has tools that help facilitate this shift in instruction by providing guidance on the 8 Effective Teaching Practices and productive vs. unproductive beliefs. 

• NCTM Principles to Actions Book and Toolkit
• NCTM Taking Action Series is an application-based approach to the publication Principles to Actions mentioned above. It includes classroom videos, transcripts, and discussion questions that can be used with teachers to help them anchor their discussions in observation.

Other great resources for districts in planning for systemic, sustained change include:

• Curriculum Support Guide from Curriculum Partners 
• Systems for Instructional Improvement: Creating Coherence from the Classroom to the District Office Creating Coherence from the Classroom to the District Office by Cobb et al.

This related IM Certified Blog post might also be helpful:

• Supporting Teachers During Implementation of Illustrative Mathematics: Big Ideas For Coaches and Teacher Leaders

We also offer an entire IM Certified Professional Learning catalog that has options for Leading IM Implementation and a menu of offerings for teachers and coaches to engage in professional learning with opportunities prior to implementation that focus on instructional routines. If you are interested in learning more about IM Certified Professional Learning opportunities, you can reach out to one of our IM Certified Partners. 

Q: As coaches, how can we facilitate simulation experiences for teachers that focus on coherence during PL sessions?

A: We offer an entire IM Certified Professional Learning catalog that has options for facilitating teacher collaboration for coaches. Coaching options include Unit-level Planning Guides. The Unit Planning Guides are asynchronous documents that coaches and PLC leaders can use to guide teachers through the initial exposure and planning for a new unit. Unit 1 sample materials are available.

If you are interested in learning more about IM Certified Professional Learning opportunities, you can reach out to one of our IM Certified Partners. 

Q: How can I best encourage current teachers to implement with fidelity and not think they need supplemental materials?

A: The best way to position teachers so that they trust the curriculum is to begin with a shared vision for teaching and learning mathematics that creates understanding of shifts in pedagogy, and then makes connections to how the curriculum supports system-wide changes in classroom practice. A common area of concern for teachers is access to the high-rigor activities of the problem-based approach and how to address unfinished learning and may be a reason for seeking supplemental materials. Professional learning affirms the belief that all students—each with unique knowledge and needs—enter the mathematics learning community as capable learners of meaningful mathematics. In professional learning sessions, educators increase their understanding of how to leverage the problem-based approach for equitable access to grade-level mathematics for all learners. The problem-based approach in the curriculum creates opportunities for access to grade-level mathematics, but it is the teacher’s facilitation moves and students’ interactions that make authentic mathematical ideas accessible to all students and cultivate a sense of pride that empowers students’ developing math identities. Instead of using supplemental materials, we recommend that teachers consider asking these questions during planning:

• Where is there opportunity to enhance access and challenge already written into the lesson?
• What can I highlight or amplify?
• What questions can I ask to build on students’ understanding?
• What tools can I offer to help students make connections to the new work?

Q: How do I help other teachers implement this curriculum in high school math classrooms?

A: As a mathematics teacher, there is power in sharing our own experiences implementing the curriculum as well as data regarding student success. We have recently published several case studies that might help start the conversation. If you are teaching IM 9–12 Math^TM in your classroom, consider inviting other teachers to visit and observe students as they interact with the lessons and each other. If it isn’t possible for teachers to observe in real time because of scheduling, consider recording a lesson on video, viewing it together, and reflecting on how the lesson supported student thinking.

Q: What is the recommended balance of hands on and digital practice for optimum learning outcomes?

A: Like many aspects of instruction, this is a personal decision based on district guidelines, the unique experiences and needs of students, and individual teacher preference. 

Q: How can we use it unplugged? What are effective ways to use the units and maintain student engagement?

A: All activities and lesson components in IM K–12 Math^TM can be used with students using print materials and do not require a device. Many districts find it useful to use engagement strategies and techniques they already have in place for other content areas, such as structures for student interaction and grouping. 

The curriculum materials include classroom structures and routines that support students in taking risks, engaging in mathematical discourse, productively struggling through problems, and participating in ways that make their ideas visible. It is through these classroom structures and routines that teachers will have daily opportunities to learn about and leverage their students’ understandings and experiences and how to position each student as a capable learner of mathematics. The collaborative learning embedded in the IM instructional model gives students an opportunity to share what they bring to the classroom. Engaging as a community of math learners builds students’ sense of belonging and self-efficacy, which creates a desire to contribute and engage in the learning experience. 

Q: Can you make some recommendations for creating a positive environment for online schools?

A: Both online and in seated classrooms, an expectation is that teachers will start off the school year establishing norms and building a mathematical community where all learners are engaged in thinking, learning, and communicating their mathematical ideas. In a mathematical community, all students have the opportunity to express their mathematical ideas and discuss them with others, which encourages collective learning and high engagement. The curriculum is a resource to support equitable structures and practices, which ensure all students have access to grade-level content and provides teachers with guidance to listen to, learn from, and support each student.

Some IM Certified blog posts that you might find helpful regarding distance learning include:

• Together Apart: Amplifying the “Gift of Student Thinking” in Distance Learning with IM
• Revisiting Distance Learning with IM K–12 Math™
• Helping Elementary Students Cultivate a Strong Math Community
• Facilitating the “Choral Counting” Routine Online

Q: In the ideal scenario, how much time should a school allocate towards this math curriculum each day?

A: IM K–5 Math^TM lessons are designed for 60 minutes of instruction. Centers are embedded in the pacing and lesson structure of K–1 and show up as optional center days in Grade 2. Grades 3–5 will need to find additional time within the school day or add in center days into the pacing. IM K–5 Math^TM also offers optional section-level practice problems that fall outside of the 60-minute block

IM 6–12 Math^TM lessons are designed for 45 minutes of instruction. IM 6–12 Math^TM also contains optional practice problems for each lesson that fall outside of the 45-minute block.

Q: Is there intervention that aligns with IM?

A: IM is designed to be used with the entire, inclusive classroom and includes built-in resources for teachers to address the needs of individual students. Many schools find that, when using IM K–12 Math, students are able to stay within the classroom and make significant progress on grade-level content without being pulled out for intervention groups. It’s preferable to keep students in the least restrictive environment, learning grade-level content alongside their peers as much as possible. 

Realizing that there may be times when unfinished learning is going to impact students’ ability to access grade-level content, the IM Team created K–5 Unit Adaptation Packs and 6–12 Unit Adaptation Packs. This teacher resource identifies ways to address gaps in prior learning and thus increase students’ access to grade-level mathematics. This blog post, Looking to the Fall Part 2: Creating a Supportive Resource for K–5 Teachers, shares more information about these manageable and useful resources for teachers.

Q: Do you have tips for balancing multi-age classrooms, 5/6 and 7/8.
Q: We are a multi-age school. We are looking for ideas about using IM in situations with two grades, especially Kindergarten and grade 1.
Q: How would you schedule a multi-age classroom?

A: The IM Certfied blog post Multi-grade Classrooms and IM K–5 Math provides some helpful insights on planning for multi-age classrooms.

Q: How do I modify the IM curriculum to give all students the ability to show their mathematical thinking?

A: IM K–12 Math is designed to elicit student thinking in the purposeful design of the tasks. When coupled with the instructional routine the Five Practices, students are provided an opportunity to show their mathematical thinking and connect their thinking and learning to those of other students. Here are some blog posts that you may find helpful:

• Adapting Problems to Elicit Student Thinking,
• Building a Mathematical Community with IM K–5 Math
• The Five Practices: Looking at Differentiation Through a New Lens

Q: What are ways to support/develop student reasoning skills in Grades K–5?

A: Problem solving provides opportunities for students to notice, wonder, estimate, pose problems, create representations, assess reasonableness, and continually make revisions as needed. A teacher’s facilitation moves can leverage students’ experiences with noticing and wondering to build students’ capacity to reason abstractly and quantitatively (MP2) and to model with mathematics (MP4). In K–5, modeling with mathematics is problem solving. In the early grades, these opportunities involve various precursor modeling skills that support students in being flexible about the way they solve problems. In upper elementary, these precursor skills become various stages of the modeling process that students will experience in grades 6–12. In addition to the precursor skills and modeling stages that appear across lessons, each unit culminates with a lesson that explicitly addresses these modeling skills and stages while pulling together the mathematical work of the unit.

Q: How does the curriculum provide both conceptual and abstract problem types for students? 

A: Curriculum design principles include a progression from more concrete to more abstract representations and also introduce new contexts or language. Activities that give all students experience with a new context ensure that students are ready to make sense of the concrete before encountering the abstract. For example, as their first encounter with a constant speed context in grade 6, students move a pre-measured distance at a constant speed and time each other (or watch a demonstration of this).

Activities that introduce a new concept and associated language build on what students already know and ask them to notice or put words to something new. For example, in grade 8, students use a picture of a figure and the same figure transformed to describe the move as, for example, a “quarter turn.” Over the course of later activities, they formalize the idea of a rotation about a point by a specific angle.

In Grades K–2, the curriculum supports students in developing meanings for addition and subtraction as they solve these problem types and they learn a progression of more concrete to more abstract methods that rely on properties of operations.

Q: How does the curriculum support opportunities for academic discourse?

A: One of the instructional routines used in IM K–12 Math^TM is the 5 Practices for Orchestrating Productive Mathematics Discussions, which are Anticipate, Monitor, Select, Sequence, and Connect. In this routine, the teacher purposefully monitors for, chooses, and sequences different student strategies to help students make connections between them and the learning goal. Curriculum activities with this routine as well as other activities offer guidance for teachers in planning for and facilitating mathematical discourse.

Q: Do you have a progression or string of tasks to demonstrate strands of content? CCSS Progressions documents have been so helpful!

A: IM K–12 Math^TM provides a coherent, standards-aligned curriculum. Our unit dependency diagrams are a great way to see how our units build within and across grades. These unit dependency charts can be found for each grade level within the course guide. IM K–5 Math^TM also offers Section Dependency Charts that can be viewed on the IM Resource Hub.

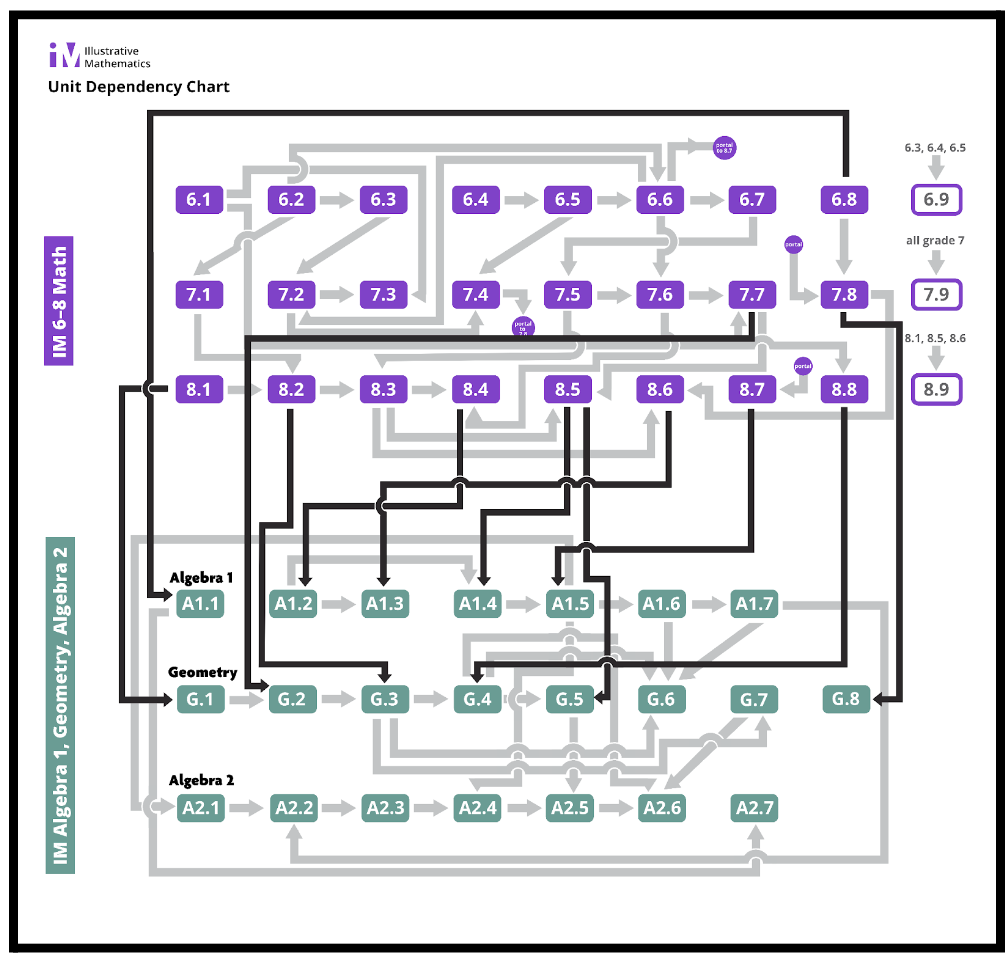
IM K–12 Math^TM is an educative curriculum meaning that we have embedded supports in the form of narratives at the unit, section, and lesson level to help teachers understand the standards that lessons are building on, addressing, and building towards.

Q: How does small-group work (with a teacher) fit into the flow of the lessons?

A: When small groups of students work in isolation of their peers to synthesize learning, it can be an equity issue and create more barriers for historically marginalized students to access grade-level mathematical meaning-making.

If we believe that all students deserve access to grade-level mathematics by engaging in the problem-based teaching and learning cycle, and we also believe that during this cycle students voice their thinking around mathematical ideas and use language to negotiate meaning with their peers. If the teacher is supported to make use of those ideas to meet the mathematical goals of the lessons, then access to grade-level mathematics means students have access to the thinking of all of their math class peers during activity and lesson syntheses. Therefore, a structure where students work in isolated groups with or without a teacher to do math or synthesize learning is not in sync with the design principles of the curriculum or what IM believes about equity.

Q: Is there a printed version of Algebra 1, Geometry, and Algebra 2 in Spanish?

A: The Algebra 1 Course and Algebra 1 Support Materials have been translated into Spanish (available from Certified Partners Kendall Hunt, and  Imagine Learning). As a nonprofit organization, Illustrative Mathematics depends on our partners or grant-funding for new initiatives, products, and services. We’re very aware of the need and it’s on our roadmap to translate all of K–12 Math into Spanish when funding becomes available.

Q: How long does it take to build this type of learning environment if the teacher is new to the idea?

A: Implementation success increases when teachers and school leaders all share a vision for mathematics teaching and learning, understand what this may mean in terms of their practice, and understand why and how adopting IM is a part of that vision. Many schools find it helpful to begin by having a discussion regarding what your district vision is for math teaching and learning. The National Council of Teachers of Mathematics (NCTM) has tools that help facilitate this shift in instruction by providing guidance on the 8 Effective Teaching Practices and productive vs. unproductive beliefs. 

• NCTM Principles to Actions Book and Toolkit
• NCTM Taking Action Series is an application based approach to the publication Principles to Actions mentioned above. It includes classroom videos, transcripts, and discussion questions that can be used with teachers to help them anchor their discussions in observation.

Professional learning and district support for school leaders will support their understanding of their role in the implementation process—alignment to vision, what to expect, how to support teacher learning, school conditions, etc. The IM Certified Professional Learning catalog has options for Leading IM implementation. Other great resources for districts in planning for systemic, sustained change include:

• Curriculum Support Guide from Curriculum Partners
• Systems for Instructional Improvement: Creating Coherence from the Classroom to the District Office Creating Coherence from the Classroom to the District Office by Cobb et al.

In addition to professional learning for leaders, the IM Certified Professional Learning Catalog includes options for teachers and coaches to engage in professional learning around our problem-based design. The instructional routines are a great place to start with teachers. If possible, some professional learning and practice with instructional routines the spring before they implement IM will give teachers a chance to try out some of the routines before implementing IM lessons. The routines are bite-sized activities that mirror the problem-based lesson structure, and include the instructional practices embedded in the curriculum. If you are interested in learning more about IM Certified Professional Learning opportunities, you can reach out to one of our IM Certified Partners. 

These related IM Certified Blog posts might also be helpful:

• Beyond Curriculum Adoption: A Vision of the IM Classroom
• Promoting Change: Reflections from the UnboundEd Five-Day Standards Institute™ 2022
• Supporting Teachers During Implementation of Illustrative Mathematics: Big Ideas For Coaches and Teacher Leaders

Q: How does IM integrate the 5 Practices for Orchestrating Productive Mathematics Discussions?

A: Promoting productive and meaningful conversations between students and teachers is essential to success in a problem-based classroom. The Instructional Routines section of the teacher course guide describes the framework presented in 5 Practices for Orchestrating Productive Mathematical Discussions (Smith & Stein, 2011) and points teachers to the book for further reading. In all lessons, teachers are supported in the practices of anticipating, monitoring, and selecting student work to share during whole-group discussions. In lessons in which there are opportunities for students to make connections between representations, strategies, concepts, and procedures, the lesson and activity narratives provide support for teachers to also use the practices of sequencing and connecting, and the lesson is tagged so teachers can easily identify these opportunities. Teachers have opportunities in curriculum workshops and PLCs to practice and reflect on their own enactment of the 5 Practices.

Q: Many IM lessons require background knowledge that students lack. What are low-lift strategies that still allow access for all?

A: IM K–5 Math is a culturally responsive curriculum in which students bring their identity (who they are) and what they know. Warm-up activities spark curiosity and help create a class culture. All activities were written with the intent to balance access and challenge.

The warm-ups consist of instructional routines that are considered our invitation to the lesson. The instructional routines are also one of our IM Certified Professional Learning courses offered in preparation for IM implementation. This IM Certified Blog post The 5 Practices Framework: Explicit Planning vs Explicit Teaching highlights an instructional routine in which teachers anticipate, monitor, select, sequence, and connect learning. This series of IM Certified Blog posts provides further insights for teachers into ways to support students as they become part of a problem-solving community of learners.

• How Do Students Perceive Problem-Based Learning?
• Inviting Students to the Mathematics
• Concrete Representations that Give Students a Way to Get Started
• Explicit Classroom Norms to Teach Kids How to Learn From Solving Problems

The IM demo site has more information about math language routines and other instructional routines. (Visit our current Certified Partners’ sites—Kendall Hunt, Imagine Learning, or McGraw-Hill—for the full curriculum.)

Q: How do you balance the curriculum components against time constraints and unfinished learning?
Q: How should one deal with a large range of math prerequisite knowledge, from grade 6 to grade 11 level?
Q: How do you adjust for students who are missing background knowledge or previous grades’ standards?
Q: How do I help those students who are 2+ years behind?

A: The authoring team created Curriculum Adaptation Packs that are housed on our IM Resource Hub to help identify prior concepts and skills that students need to access the content in each unit. These adaptation packs also provide just-in-time support to keep students progressing in their learning.

Q: What is IM’s future plan for additional intervention materials, especially for students who are experiencing IM for the first time?

A: IM is designed to be used with the entire, inclusive classroom with built-in teaching moves. This approach is in contrast to a strategy of “teaching to the middle” and then intervening, using separate programs, for students who aren’t getting what they need. Individual students can have their unique needs addressed within the structure of the IM lessons and centers. When a teacher is responding to student work during the lesson, each student’s learning is being addressed just in time and not postponed until later with a separate experience.